It was frequently mentioned in the preceding chapter that musical tones could be resolved by the ear alone, unassisted by any peculiar apparatus, into a series of partial tones corresponding to the simple pendular vibrations in a mass of air, that is, into the same constituents as those into which the motion of the air is resolved by the sympathetic vibration of elastic bodies. We proceed to shew the correctness of this assertion.
Any one who endeavours for the first time to distinguish the upper partial tones of a musical tone, generally finds considerable difficulty in merely hearing them.
The analysis of our sensations when it cannot be attached to corresponding differences in external objects, meets with peculiar difficulties, the nature and significance of which will have to be considered hereafter. The attention of the observer has generally to be drawn to the phenomenon he has to observe, by peculiar aids properly selected, until he knows precisely what to look for; after he has once succeeded, he will be able to throw aside such crutches. Similar difficulties meet us in the observation of the upper partials of a musical tone. I shall first give a description of such processes as will most easily put an untrained observer into a position to recognise upper partial tones, and I will remark in passing that a musically trained ear will not necessarily hear upper partial tones with greater ease and certainty than an untrained ear. Success depends rather upon a peculiar power of mental abstraction or a peculiar mastery over attention, than upon musical training. But a musically trained observer has an essential advantage over one not so trained in his power of figuring to himself how the simple tones sought for ought to sound, whereas the untrained observer has continually to hear these tones sounded by other means in order to keep their effect fresh in his mind.
First we must note, that the unevenly numbered partials, as the Fifths, Thirds, Sevenths, &c., of the prime tones, are usually easier to hear than the even ones, which are Octaves either of the prime tone or of some of the upper partials which lie near it, just as in a chord we more readily distinguish whether it contains Fifths and Thirds than whether it has Octaves. The second, fourth, and eighth partials are higher Octaves of the prime, the sixth partial an Octave above the third partial, that is, the Twelfth of the prime; and some practice is required for distinguishing these. Among the uneven partials which are more easily distinguished, the first place must be assigned, from its usual loudness, to the third partial, the Twelfth of the prime, or the Fifth of its first higher Octave. Then follows the fifth partial as the major Third of the prime, and, generally very faint, the seventh partial as the minor Seventh [1] of the second higher Octave of the prime, as will be seen by their following expression in musical notation, for the compound tone \(c\).
In commencing to observe upper partial tones, it is advisable just before producing the musical tone itself which you wish to analyse, to sound the note you wish to distinguish in it, very gently, and if possible in the same quality of tone as the compound itself. The pianoforte and harmonium are well adapted for these experiments, because they both have upper partial tones of considerable power.
First gently strike on a piano the note \(g'\), as marked above, and after letting the digital [3] rise so as to damp the string, Strike the note \(c\), of which \(g'\) is the third partial, with great force, and keep your attention directed to the pitch of the \(g'\) which you had just heard, and you will hear it again in the compound tone of \(c\). Similarly, first strike the fifth partial \(e''\) gently and then \(c\) strongly. These upper partial tones are often more distinct as the sound dies away, because they appear to lose force more slowly than the prime. The seventh and ninth partials \(b''\flat\) and \(d'''\) are mostly weak, or quite absent on modern pianos. If the same experiments are tried with an harmonium in one of its louder stops, the seventh partial will generally be well heard, and sometimes even the ninth.
To the objection which is sometimes made that the observer only imagines he hears the partial tone in the compound, because he had just heard it by itself, I need only remark at present that if \(e''\) is first heard as a partial tone of \(c\) on a good piano, tuned in equal temperament, and then \(e''\) is struck on the instrument itself, it is quite easy to perceive that the latter is a little sharper. This follows from the method of tuning. But if there is a difference in pitch between the two tones, one is certainly not a continuation of the mental effect produced by the other. Other facts which completely refute the above conception, will be subsequently adduced.
A still more suitable process than that just described for the piano, can be adopted on any stringed instrument, as the piano, monochord, or violin. It consists in first producing the tone we wish to hear, as an harmonic [p. 25, note] by touching the corresponding node of the string when it is struck or rubbed. The resemblance of the tone first heard to the corresponding partial of the compound is then much greater, and the ear discovers it more readily. It is usual to place a divided scale by the string of a monochord, to facilitate the discovery of the nodes. Those for the third partial, as shewn in Chap. III. (p. 45), divide the string into three equal parts, those for the fifth into five, and so on. On the piano and violin the position of these points is easily found experimentally, by touching the string gently with the finger in the neighbourhood of the node, which has been approximatively determined by the eye, then striking or bowing the string, and moving the finger about till the required harmonic comes out strongly and purely. By then sounding the string, at one time with the finger on the node, and at another without, we obtain the required upper partial at one time as an harmonic, and at another in the compound tone of the whole string, and thus learn to recognise the existence of the first as part of the second, with comparative ease. Using thin strings which have loud upper partials, I have thus been able to recognise the partials separately, up to the sixteenth. Those which lie still higher are too near to each other in pitch for the ear to separate them readily.
In such experiments I recommend the following process. Touch the node of the string on the pianoforte or monochord with a camel's hair pencil, strike the note, and immediately remove the pencil from the string. If the pencil has been pressed tightly on the string, we either continue to hear the required partial as an harmonic, or else in addition hear the prime tone gently sounding with it. On repeating the excitement of the string, and continuing to press more and more lightly with the camel's-hair pencil, and at last removing the pencil entirely, the prime tone of the string will be heard more and more distinctly with the harmonic till we have finally the full natural musical tone of the string. By this means we obtain a series of gradual transitional stages between the isolated partial and the compound tone, in which the first is readily retained by the ear. By applying this last process I have generally succeeded in making perfectly untrained ears recognise the existence of upper partial tones.
It is at first more difficult to hear the upper partials on most wind instruments and in the human voice, than on stringed instruments, harmoniums, and the more penetrating stops of an organ, because it is then not so easy first to produce the upper partial softly in the same quality of tone. But still a little practice suffices to lead the ear to the required partial tone, by previously touching it on the piano. The partial tones of the human voice are comparatively most difficult to distinguish for reasons which will be given subsequently. Nevertheless they were distinguished even by Rameau [4] without the assistance of any apparatus. The process is as follows:—
Get a powerful bass voice to sing \(e\flat\) to the vowel O, in sore [more like aw in saw than o in so], gently touch \(b'\flat\) on the piano, which is the Twelfth, or third partial tone of the note \(e\flat\), and let its sound die away while you are listening to it attentively. The note \(b'\flat\) on the piano will appear really not to die away, but to keep on sounding, even when the string is damped by removing the finger from the digital, because the ear unconsciously passes from the tone of the piano to the partial tone of the same pitch produced by the singer, and takes the latter for a continuation of the former. But when the finger is removed from the key, and the damper has fallen, it is of course impossible that the tone of the string should have continued sounding. To make the experiment for \(g''\) the fifth partial, or major Third of the second Octave above \(e\flat\), the voice should sing to the vowel A in farther.
The resonators described in the last chapter furnish an excellent means for this purpose, and can be used for the tones of any musical instrument. On applying to the ear the resonator corresponding to any given upper partial of the compound \(c\), such as \(g'\), this \(g'\) is rendered much more powerful when \(c\) is sounded. Now hearing and distinguishing \(g'\) in this case by no means proves that the ear alone and without this apparatus would hear \(g'\) as part of the compound \(c\). But the increase of the loudness of \(g'\) caused by the resonator may be used to direct the attention of the ear to the tone it is required to distinguish. On gradually removing the resonator from the ear, the force of \(g'\) will decrease. But the attention once directed to it by this means, remains more readily fixed upon it, and the observer continues to hear this tone in the natural and unchanged compound tone of the given note, even with his unassisted ear. The sole office of the resonators in this case is to direct the attention of the ear to the required tone.
By frequently instituting similar experiments for perceiving the upper partial tones, the observer comes to discover them more and more easily, till he is finally able to dispense with any aids. But a certain amount of undisturbed concentration is always necessary for analysing musical tones by the ear alone, and hence the use of resonators is quite indispensable for an accurate comparison of different qualities of tone, especially in respect to the weaker upper partials. At least, I must confess, that my own attempts to discover the upper partial tones in the human voice, and to determine their differences for different vowels, were most unsatisfactory until I applied the resonators.
We now proceed to prove that the human ear really does analyse musical tones according to the law of simple vibrations. Since it is not possible to institute an exact comparison of the strength of our sensations for different simple tones, we must confine ourselves to proving that when an analysis of a composite tone into simple vibrations, effected by theoretic calculation or by sympathetic resonance, shews that certain upper partial tones are absent, the ear also does not perceive them.
The tones of strings are again best adapted for conducting this proof, because they admit of many alterations in their quality of tone, according to the manner and the spot in which they are excited, and also because the theoretic or experimental analysis is most easily and completely performed for this case. Thomas Young [5] first shewed that when a string is plucked or struck, or, as we may add, bowed at any point in its length which is the node of any of its so-called harmonics, those simple vibrational forms of the string which have a node in that point are not contained in the compound vibrational form. Hence, if we attack the string at its middle point, all the simple vibrations due to the evenly numbered partials, each of which has a note at that point, will be absent. This gives the sound of the string a peculiarly hollow or nasal twang. If we excite the string at \(\tfrac{1}{3}\) of its length, the vibrations corresponding to the third, sixth, and ninth partials will be absent; if at \(\tfrac{1}{4}\), then those corresponding to the fourth, eighth, and twelfth partials will fail; and so on.[6]
This result of mathematical theory is confirmed, in the first place, by analysing the compound tone of the string by sympathetic resonance, either by the resonators or by other strings. The experiments may be easily made on the pianoforte. Press down the digitals for the note \(c\) and \(c'\), without allowing the hammer to strike, so as merely to free them from their dampers, and then pluck the string \(c\) with the nail till it sounds. On damping the \(c\) string the higher \(c'\) will echo the sound, except in the particular case when the \(c\) string has been plucked exactly at its middle point, which is the point where it would have to be touched in order to give its first harmonic when struck by the hammer.
If we touch the \(c\) string at \(\tfrac{1}{3}\) or \(\tfrac{2}{3}\) its length, and strike it with the hammer, we obtain the harmonic \(g'\); and if the damper of the \(g'\) is raised, this string echoes the sound. But if we pluck the \(c\) string with the nail, at either \(\tfrac{1}{3}\) or \(\tfrac{2}{3}\) its length, \(g'\) is not echoed, as it will be if the \(c\) string is plucked at any other spot.
In the same way observations with the resonators shew that when the \(c\) string is plucked at its middle the Octave \(c'\) is missing, and when at \(\tfrac{1}{3}\) or \(\tfrac{2}{3}\) its length the Twelfth \(g'\) is absent. The analysis of the sound of a string by the sympathetic resonance of strings or resonators, consequently fully confirms Thomas Young’s law.
But for the vibration of strings we have a more direct means of analysis than that furnished by sympathetic resonance. If we, namely, touch a vibrating string gently for a moment with the finger or a camel's-hair pencil, we damp all those simple vibrations which have no node at the point touched. Those vibrations, however, which have a node there are not damped, and hence will continue to sound without the others. Consequently, if a string has been made to speak in any way whatever, and we wish to know whether there exists among its simple vibrations one corresponding to the Twelfth of the prime tone, we need only touch one of the nodes of this vibrational form at \(\tfrac{1}{3}\) or \(\tfrac{2}{3}\) the length of the string, in order to reduce to silence all simple tones which have no such node, and leave the Twelfth sounding, if it were there. If neither it, nor any of the sixth, ninth, twelfth, &c., of the partial tones were present, giving corresponding harmonics, the string will be reduced to absolute silence by this contact of the finger.
Press down one of the digitals of a piano, in order to free a string from its damper. Pluck the string at its middle point, and immediately touch it there. The string will be completely silenced, shewing that plucking it in its middle excited none of the evenly numbered partials of its compound tone. Pluck it at \(\tfrac{1}{3}\) or \(\tfrac{2}{3}\) its length, and immediately touch it in the same place; the string will be silent, proving the absence of the third partial tone. Pluck the string anywhere else than in the points named, and the second partial will he heard when the middle is touched, the third when the string is touched at \(\tfrac{1}{3}\) or \(\tfrac{2}{3}\) of its length.
The agreement of this kind of proof with the results from sympathetic resonance, is well adapted for the experimental establishment of the proposition based in the last chapter solely upon the results of mathematical theory, namely, that sympathetic vibration occurs or not, according as the corresponding simple vibrations are or are not contained in the compound motion. In the last described method of analysing the tone of a string, we are quite independent of the theory of sympathetic vibration, and the simple vibrations of strings are exactly characterised and recognisable by their nodes. If the compound tones admitted of being analysed by sympathetic resonance according to any other vibrational forms except those of simple vibration, this agreement could not exist.
If, after having thus experimentally proved the correctness of Thomas Young's law, we try to analyse the tones of strings by the unassisted ear, we shall continue to find complete agreement.[7] If we pluck or strike a string in one of its nodes, all those upper partial tones of the compound tone of the string to which the node belongs, disappear for the ear also, but they are heard if the string is plucked at any other place. Thus, if the string \(c\) be plucked at \(\tfrac{1}{3}\) its length, the partial tone \(g'\) cannot be heard, but if the string be plucked at only a little distance from this point the partial tone \(g'\) is distinctly audible. Hence the ear analyses the sound of a string into precisely the same constituents as are found by sympathetic resonance, that is, into simple tones, according to Ohm's definition of this conception These experiments are also well adapted to shew that it is no mere play of imagination when we hear upper partial tones, as some people believe on hearing them for the first time, for those tones are not heard when they do not exist.
The following modification of this process is also very well adapted to make the upper partial tones of strings audible. First, strike alternately in rhythmical sequence, the third and fourth partial tone of the string alone, by damping it in the corresponding nodes, and request the listener to observe the simple melody thus produced. Then strike the undamped string alternately and in the same rhythmical sequence, in these nodes, and thus reproduce the same melody in the upper partials, which the listener will then easily recognise. Of course, in order to hear the third partial, we must strike the string in the node of the fourth, and conversely.
The compound tone of a plucked string is also a remarkably striking example of the power of the ear to analyse into a long series of partial tones, a motion which the eye and the imagination are able to conceive in a much simpler manner. A string, which is pulled aside by a sharp point, or the finger nail, assumes the form, fig. 18, A (p. 54), before it is released. It then passes through the series of forms, fig. 18, B, C, D, E, F, till it reaches G, which is the inversion of A, and then returns, through the same, to A again. Hence it alternates between the forms A and G. All these forms, as is clear, are composed of three straight lines, and on expressing the velocity of the individual points of the strings by vibrational curves, these would have the same form. Now the string scarcely imparts any perceptible portion of its own motion directly to the air. Scarcely any audible tone results when both ends of a string are fastened to immovable supports, as metal bridges, which are again fastened to the walls of a room. The sound of the string reaches the air through that one of its extremities which rests upon a bridge standing on an elastic sounding board. Hence the sound of the string essentially depends on the motion of this extremity, through the pressure which it exerts on the sounding board.
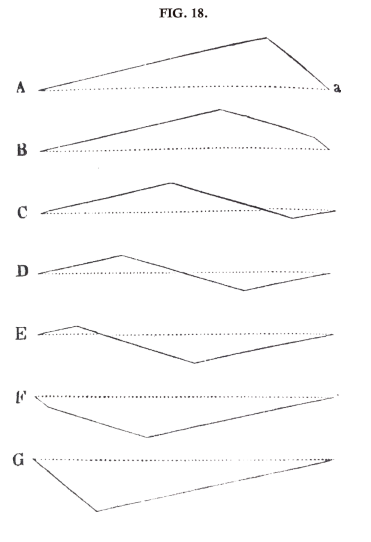
The magnitude of this pressure, as it alters periodically with the time, is shewn in fig. 19, where the height of the line h h corresponds to the amount of pressure exerted on the bridge by that extremity of the string when the string is at rest. Along h h suppose lengths to be set off corresponding to consecutive intervals of time, the vertical heights of the broken line above or below h h represent the corresponding augmentations or diminutions of pressure at those times. The pressure of the string on the sounding board consequently alternates, as the figure shews, between a higher and a lower value. For some time the greater pressure remains unaltered; then the lower suddenly ensues, and likewise remains for a time unaltered. The letters a to g in fig. 19 correspond to the times at which the string assumes the forms A to G in fig. 18. It is this alteration between a greater and a smaller pressure which produces the sound in the air. We cannot but feel astonished that a motion produced by means so simple and so easy to comprehend, should be analysed by the ear into such a complicated sum of simple tones. For the eye and the understanding the action of the string on the sounding board can be figured with extreme simplicity. What has the simple broken line of fig. 19 to do with wave-curves, which, in the course of one of their periods, shew

3, 4, 5, up to 16, and more, crests and troughs? This is one of the most striking examples of the different ways in which eye and ear comprehend a periodic motion.
This app simulates a plucked string, similar to that in a guitar. Click on the base line to simulate dampening the string in that place. The harmonics are marked with tallies.
There is no sonorous body whose motions under varied conditions can be so completely calculated theoretically and contrasted with observation as a string. The following are examples in which theory can be compared with analysis by ear:—
I have discovered a means of exciting simple pendular vibrations in the air. A tuning-fork when struck gives no harmonic upper partial tones, or, at most, traces of them when it is brought into such excessively strong vibration that it no longer exactly follows the law of the pendulum.[8] On the other hand, tuning-forks have some very high inharmonic secondary tones, which produce that peculiar sharp tinkling of the fork at the moment of being struck, and generally become rapidly inaudible. If the tuning-fork is held in the fingers, it imparts very little of its tone to the air, and cannot be heard unless it is held close to the ear. Instead of holding it in the fingers, we may screw it into a thick board, on the under side of which some pieces of india-rubber tubing have been fastened. When this is laid upon a table, the india-rubber tubes on which it is supported convey no sound to the table, and the tone of the tuning-fork is so weak that it may be considered inaudible. Now if the prongs of the fork be brought near a resonance chamber [9] of a bottle-form of such a size and shape that, when we blow over its mouth, the air it contains gives a tone of the same pitch as the fork's, the air within this chamber vibrates sympathetically, and the tone of the fork is thus conducted with great strength to the outer air. Now the higher secondary tones of such resonance chambers are also inharmonic to the prime tone, and in general the secondary tones of the chambers correspond neither with the harmonic nor the inharmonic secondary tones of the forks; this can be determined in each particular case by producing the secondary tones of the bottle by stronger blowing, and discovering those of the forks with the help of strings set into sympathetic vibration, as will be presently described. If, then, only one of the tones of the fork, namely, the prime tone, corresponds with one of the tones of the chamber, this alone will be reinforced by sympathetic vibration, and this alone will be communicated to the external air, and thus conducted to the observer's ear. The examination of the motion of the air by resonators shews that in this case, provided the tuning-fork be not set into too violent motion, no tone but the prime is present, and in such case the unassisted ear hears only a single simple tone, namely, the common prime of the tuning-fork and of the chamber, without any accompanying upper partial tones.
The tone of a tuning-fork can also be purified from secondary tones by placing its handle upon a string and moving it so near to the bridge that one of the proper tones of the section of string lying between the fork and the bridge is the same as that of the tuning-fork. The string then begins to vibrate strongly, and conducts the tone of the tuning-fork with great power to the sounding board and surrounding air, whereas the tone is scarcely, if at all, heard as long as the above-named section is not in unison with the tone of the fork. In this way it is easy to find the lengths of string which correspond to the prime and upper partial tones of the fork, and accurately determine the pitch of the latter. If this experiment is conducted with ordinary strings which are uniform throughout their length, we shield the ear from the inharmonic secondary tones of the fork, but not from the harmonic upper partials, which are sometimes faintly present when the fork is made to vibrate strongly. Hence to conduct this experiment in such a way as to create purely pendular vibrations of the air, it is best to weight one point of the string, if only so much as by letting a drop of melting sealing-wax fall upon it. This causes the upper proper tones of the string itself to be inharmonic to the prime tone, and hence there is a distinct interval between the points where the fork must be placed to bring out the prime tone and its audible Octave, if it exists.
In most other cases the mathematical analysis of the motions of sound is not nearly far enough advanced to determine with certainty what upper partials will be present and what intensity they will possess. In circular plates and stretched membranes which are struck, it is theoretically possible to do so, but their inharmonic secondary tones are so numerous and so nearly of the same pitch that most observers would probably fail to separate them satisfactorily. On elastic rods, however, the secondary tones are very distant from each other, and are inharmonic, so that they can be readily distinguished from each other by the ear. The following are the proper tones of a rod which is free at both ends; the vibrational number of the prime tone taken to be \(c\), is reckoned as 1:—
| Pitch Number | Cents [10] | Notation | |
| Prime tone | 1.0000 |
|
\(c\) |
| Second proper tone | 2.7576 |
|
\(f' \;\) + 0.2 |
| Third proper tone | 5.4041 |
|
\(f'' \;\) + 0.1 |
| Fourth proper tone | 13.3444 |
|
\(a'' \;\) -0.1 |
The notation is adapted to the equal temperament, and the appended fractions are parts of the interval of a complete tone.
Where we are unable to execute the theoretical analysis of the motion, we can, at any rate, by means of resonators and other sympathetically vibrating bodies, analyse any individual musical tone that is produced, and then compare this analysis, which is determined by the laws of sympathetic vibration, with that effected by the unassisted ear. The latter is naturally much less sensitive than one armed with a resonator; so that it is frequently impossible for the unarmed ear to recognise amongst a number of other stronger simple tones those which the resonator itself can only faintly indicate. On the other hand, so far as my experience goes, there is complete agreement to this extent: the ear recognises without resonators the simple tones which the resonators greatly reinforce, and perceives no upper partial tone which the resonator does not indicate. To verify this conclusion, I performed numerous experiments, both with the human voice and the harmonium, and they all confirmed it.[11]
By the above experiments the proposition enunciated and defended by G. S. Ohm must be regarded as proved, viz. that the human ear perceives pendular vibrations alone as simple tones, and resolves all other periodic motions of the ear into a series of pendular vibrations, hearing the series of simple tones which correspond with these simple vibrations.
Calling, then, as already defined (in pp. 23, 24 and note), the sensation excited in the ear by any periodical motion of the air a musical tone, and the sensation excited by a simple pendular vibration a simple tone, the rule asserts that the sensation of a musical tone is compounded out of the sensations of several simple tones. In particular, we shall henceforth call the sound produced by a single sonorous body its (simple or compound) tone, and the sound produced by several musical instruments acting at the same time a composite tone, consisting generally of several (simple or compound) tones. If, then, a single note is sounded on a musical instrument, as a violin, trumpet, organ, or by a singing voice, it must be called in exact language a tone of the instrument in question. This is also the ordinary language, but it did not then imply that the tone might be compound. When the tone is, as usual, a compound tone, it will be distinguished by this term, or the abridgment, a compound; while tone is a general term which includes both simple and compound tones.[12] The prime tone is generally louder than any of the upper partial tones, and hence it alone generally determines the pitch of the compound. The tone produced by any sonorous body reduces to a single simple tone in very few cases indeed, as the tone of tuning-forks imparted to the air by resonance chambers in the manner already described. The tones of wide-stopped organ pipes when gently blown are almost free from upper partials, and are accompanied only by a rush of wind.
It is well known that this union of several simple tones into one compound tone, which is naturally effected in the tones produced by most musical instruments, is artificially imitated on the organ by peculiar mechanical contrivances. The tones of organ pipes are comparatively poor in upper partials. When it is desirable to use a stop of incisive penetrating quality of tone and great power, the wide pipes (principal register and weitgedackt [13]) are not sufficient; their tone is too soft, too defective in upper partials; and the narrow-pipes (geigen-register and quintaten [14]) are also unsuitable, because, although more incisive, their tone is weak. For such occasions, then, as in accompanying congregational singing, recourse is had to the compound stops.[15] In these stops every key is connected with a larger or smaller series of pipes, which it opens simultaneously, and which give the prime tone and a certain number of the lower upper partials of the compound tone of the note in question. It is very usual to connect the upper Octave with the prime tone, and after that the Twelfth. The more complex compounds (cornet [15]) give the first six partial tones, that is, in addition to the two Octaves of the prime tone and its Twelfth, the higher major Third, and the Octave of the Twelfth. This is as much of the series of upper partials as belongs to the tones of a major chord. But to prevent these compound stops from being insupportably noisy, it is necessary to reinforce the deeper tones of each note by other rows of pipes, for in all natural tones which are suited for musical purposes the higher partials decrease in force as they rise in pitch. This has to be regarded in their imitation by compound stops. These compound stops were a monster in the path of the old musical theory; which was acquainted only with the prime tones of compounds; but the practice of organ-builders and organists necessitated their retention, and when they are suitably arranged and properly applied, they form a very effective musical apparatus.
The nature of the case at the same time fully justifies their use. The musician is bound to regard the tones of all musical instruments as compounded in the same way as the compound stops of organs, and the important part this method of composition plays in the construction of musical scales and chords will be made evident in subsequent chapters.
We have thus been led to an appreciation of upper partial tones, which differs considerably from that previously entertained by musicians, and even physicists, and must therefore be prepared to meet the opposition which will be raised. The upper partial tones were indeed known, but almost only in such compound tones as those of strings, where there was a favourable opportunity for observing them; but they appear in previous physical and musical works as an isolated accidental phenomenon of small intensity, a kind of curiosity, which was certainly occasionally adduced, in order to give some support to the opinion that nature had prefigured the construction of our major chord, but which on the whole remained almost entirely disregarded. In opposition to this we have to assert, and we shall prove the assertion in the next chapter, that upper partial tones are, with a few exceptions already named, a general constituent of all musical tones, and that a certain stock of upper partials is an essential condition for a good musical quality of tone. Finally, these upper partials have been erroneously considered as weak, because they are difficult to observe, while, in point of fact, for some of the best musical qualities of tone, the loudness of the first upper partials is not far inferior to that of the prime tone itself.
There is no difficulty in verifying this last fact by experiments on the tones of strings. Strike the string of a piano or monochord, and immediately touch one of its nodes for an instant with the finger; the constituent partial tones having this node will remain with unaltered loudness, and the rest will disappear. We might also touch the node in the same way at the instant of striking, and thus obtain the corresponding constituent partial tones from the first, in place of the complete compound tone of the note. In both ways we can readily convince ourselves that the first upper partials, as the Octave and Twelfth, are by no means weak and difficult to hear, but have a very appreciable strength. In some cases we are able to assign numerical values for the intensity of the upper partial tones, as will be shewn in the next chapter. For tones not produced on strings this à posteriori proof is not so easy to conduct, because we are not able to make the upper partials speak separately. But even then by means of the resonator we can appreciate the intensity of these upper partials by producing the corresponding note on the same or some other instrument until its loudness, when heard through the resonator, agrees with that of the former.
The difficulty we experience in hearing upper partial tones is no reason for considering them to be weak; for this difficulty does not depend on their intensity, but upon entirely different circumstances, which could not be properly estimated until the advances recently made in the physiology of the senses. On this difficulty of observing the upper partial tones have been founded the objections which A. Seebeck [16] has advanced against Ohm's law of the decomposition of a musical tone; and perhaps many of my readers who are unacquainted with the physiology of the other senses, particularly with that of the eye, might be inclined to adopt Seebeck's opinions. I am therefore obliged to enter into some details concerning this difference of opinion, and the peculiarities of the perceptions of our senses, on which the solution of the difficulty depends.
Seebeck, although extremely accomplished in acoustical experiments and observations, was not always able to recognise upper partial tones, where Ohm's law required them to exist. But we are also bound to add that he did not apply the methods already indicated for directing the attention of his ear to the upper partials in question. In other cases when he did hear the theoretical upper partials, they were weaker than the theory required. He concluded that the definition of a simple tone as given by Ohm was too limited, and that not only pendular vibrations, but other vibrational forms, provided they were not too widely separated from the pendular, were capable of exciting in the ear the sensation of a single simple tone, which, however, had a variable quality. He consequently asserted that when a musical tone was compounded of several simple tones, part of the intensity of the upper constituent tones went to increase the intensity of the prime tone, with which it fused, and that at most a small remainder excited in the ear the sensation of an upper partial tone. He did not formulate any determinate law, assigning the vibrational forms which would give the impression of a simple and those which would give the impression of a compound tone. The experiments of Seebeck, on which he founded his assertions, need not be here described in detail. Their object was only to produce musical tones for which either the intensity of the simple vibrations corresponding to the upper partials could be theoretically calculated, or in which these upper partials could be rendered separately audible. For the latter purpose the siren was used. We have just described how the same object can be attained by means of strings. Seebeck shews in each case that the simple vibrations corresponding to the upper partials have considerable strength, but that the upper partials are either not heard at all, or heard with difficulty in the compound tone itself. This fact has been already mentioned in the present chapter. It may be perfectly true for an observer who has not applied the proper means for observing upper partials, while another, or even the first observer himself when properly assisted, can hear them perfectly well.[17]
Now there are many circumstances which assist us first in separating the musical tones arising from different sources, and secondly, in keeping together the partial tones of each separate source. Thus when one musical tone is heard for some time before being joined by the second, and then the second continues after the first has ceased, the separation in sound is facilitated by the succession of time. We have already heard the first musical tone by itself, and hence know immediately what we have to deduct from the compound effect for the effect of this first tone. Even when several parts proceed in the same rhythm in polyphonic music, the mode in which the tones of different instruments and voices commence, the nature of their increase in force, the certainty with which they are held, and the manner in which they die off, are generally slightly different for each. Thus the tones of a pianoforte commence suddenly with a blow, and are consequently strongest at the first moment, and then rapidly decrease in power. The tones of brass instruments, on the other hand, commence sluggishly, and require a small but sensible time to develop their full strength. The tones of bowed instruments are distinguished by their extreme mobility, but when either the player or the instrument is not unusually perfect they are interrupted by little, very short, pauses, producing in the ear the sensation of scraping, as will be described more in detail when we come to analyse the musical tone of a violin. When, then, such instruments are sounded together there are generally points of time when one or the other is predominant, and it is consequently easily distinguished by the ear. But besides all this, in good part music, especial care is taken to facilitate the separation of the parts by the ear. In polyphonic music proper, where each part has its own distinct melody, a principal means of clearly separating the progression of each part has always consisted in making them proceed in different rhythms and on different divisions of the bars; or where this could not be done, or was at any rate only partly possible, as in four-part chorales, it is an old rule, contrived for this purpose, to let three parts, if possible, move by single degrees of the scale, and let the fourth leap over several. The small amount of alteration in the pitch makes it easier for the listener to keep the identity of the several voices distinctly in mind.
Here we have a function generator which allows us to sketch a function. We have a set of springs tuned to the first 5 harmonics of the sketched function.
All these helps fail in the resolution of musical tones into their constituent partials. When a compound tone commences to sound, all its partial tones commence with the same comparative strength; when it swells, all of them generally swell uniformly; when it ceases, all cease simultaneously. Hence no opportunity is generally given for hearing them separately and independently. In precisely the same manner as the naturally connected partial tones form a single source of sound, the partial tones in a compound stop on the organ fuse into one, as all are struck with the same digital, and all move in the same melodic progression as their prime tone.
Moreover, the tones of most instruments are usually accompanied by characteristic irregular noises, as the scratching and rubbing of the violin bow, the rush of wind in flutes and organ pipes, the grating of reeds, &c. These noises, with which we are already familiar as characterising the instruments, materially facilitate our power of distinguishing them in a composite mass of sounds. The partial tones in a compound have, of course, no such characteristic marks.
Hence we have no reason to be surprised that the resolution of a compound tone into its partials is not quite so easy for the ear to accomplish, as the resolution of composite masses of the musical sounds of many instruments into their proximate constituents, and that even a trained musical ear requires the application of a considerable amount of attention when it undertakes the former problem.

It is easy to see that the auxiliary circumstances already named do not always suffice for a correct separation of musical tones. In uniformly sustained musical tones, where one might be considered as an upper partial of another, our judgment might readily make default. This is really the case. G. S. Ohm proposed a very instructive experiment to shew this, using the tones of a violin. But it is more suitable for such an experiment to use simple tones, as those of a stopped organ pipe. The best instrument, however, is a glass bottle of the form shewn in fig. 20, which is easily procured and prepared for the experiment. A little rod c supports a guttapercha tube a in a proper position. The end of the tube, which is directed towards the bottle, is softened in warm water and pressed flat, forming a narrow chink, through which air can be made to rush over the mouth of the bottle. When the tube is fastened by an india-rubber pipe to the nozzle of a bellows, and wind is driven over the bottle, it produces a hollow obscure sound, like the vowel oo in too, which is freer from upper partial tones than even the tone of a stopped pipe, and is only accompanied by a slight noise of wind. I find that it is easier to keep the pitch unaltered in this instrument while the pressure of the wind is slightly changed, than in stopped pipes. We deepen the tone by partially shading the orifice of the bottle with a little wooden plate; and we sharpen it by pouring in oil or melted wax. We are thus able to make any required little alterations in pitch. I tuned a large bottle to \(b\flat\) and a smaller one to \(b'\flat\) and united them with the same bellows, so that when used both began to speak at the same instant. When thus united they gave a musical tone of the pitch of the deeper \(b\flat\) but having the quality of tone of the vowel oa in toad, instead of oo in too. When, then, I compressed first one of the india-rubber tubes and then the other, so as to produce the tones alternately, separately, and in connection, I was at last able to hear them separately when sounded together, but I could not continue to hear them separately for long, for the upper tone gradually fused with the lower. This fusion takes place even when the upper tone is somewhat stronger than the lower. The alteration in the quality of tone which takes place during this fusion is characteristic. On producing the upper tone first and then letting the lower sound with it, I found that I at first continued to hear the upper tone with its full force, and the under tone sounding below it in its natural quality of oo in too. But by degrees, as my recollection of the sound of the isolated upper tone died away, it seemed to become more and more indistinct and weak, while the lower tone appeared to become stronger, and sounded like oa in toad. This weakening of the upper and strengthening of the lower tone was also observed by Ohm on the violin. As Seebeck remarks, it certainly does not always occur, and probably depends on the liveliness of our recollections of the tones as heard separately, and the greater or less uniformity in the simultaneous production of the tones. But where the experiment succeeds, it gives the best proof of the essential dependence of the result on varying activity of attention. With the tones produced by bottles, in addition to the reinforcement of the lower tone, the alteration in its quality is very evident and is characteristic of the nature of the process. This alteration is less striking for the penetrating tones of the violin.[18]
This experiment has been appealed to both by Ohm and by Seebeck as a corroboration of their different opinions. When Ohm stated that it was an 'illusion of the ear' to apprehend the upper partial tones wholly or partly as a reinforcement of the prime tone (or rather of the compound tone whose pitch is determined by that of its prime), he certainly used a somewhat incorrect expression, although he meant what was correct, and Seebeck was justified in replying that the ear was the sole judge of auditory sensations, and that the mode in which it apprehended tones ought not to be called an 'illusion'. However, our experiments just described shew that the judgment of the ear differs according to the liveliness of its recollection of the separate auditory impressions here fused into one whole, and according to the intensity of its attention. Hence we can certainly appeal from the sensations of an ear directed without assistance to external objects, whose interests Seebeck represents, to the ear which is attentively observing itself and is suitably assisted in its observation. Such an ear really proceeds according to the law laid down by Ohm.
Another experiment should be adduced. Raise the dampers of a pianoforte so that all the strings can vibrate freely, then sing the vowel a in father, art, loudly to any note of the piano, directing the voice to the sounding-board; the sympathetic resonance of the strings distinctly re-echoes the same a. On singing oe in toe, the same oe is re-echoed. On singing a in fare, this a is re-echoed. For ee in see the echo is not quite so good. The experiment does not succeed so well if the damper is removed only from the note on which the vowels are sung. The vowel character of the echo arises from the re-echoing of those upper partial tones which characterise the vowels. These, however, will echo better and more clearly when their corresponding higher strings are free and can vibrate sympathetically. In this case, then, in the last resort, the musical effect of the resonance is compounded of the tones of several strings, and several separate partial tones combine to produce a musical tone of a peculiar quality. In addition to the vowels of the human voice, the piano will also quite distinctly imitate the quality of tone produced by a clarinet, when strongly blown on to the sounding board.
Finally, we must remark, that although the pitch of a compound tone is, for musical purposes, determined by that of its prime, the influence of the upper partial tones is by no means unfelt. They give the compound tone a brighter and higher effect. Simple tones are dull. When they are compared with compound tones of the same pitch, we are inclined to estimate the compound as belonging to a higher Octave than the simple tones. The difference is of the same kind as that heard when first the vowel oo in too and then a in tar are sung to the same note. It is often extremely difficult to compare the pitches of compound tones of different qualities. It is very easy to make a mistake of an Octave. This has happened to the most celebrated musicians and acousticians. Thus it is well known that Tartini, who was celebrated as a violinist and theoretical musician, estimated all combinational tones (Chap. XI.) an Octave too high, and, on the other hand, Henrici [19] as well known to musicians assigns a pitch too low by an Octave to the upper partial tones of tuning-forks.[20]
The problem to be solved, then, in distinguishing the partials of a compound tone is that of analysing a given aggregate of sensations into elements which no longer admit of analysis. We are accustomed in a large number of cases where sensations of different kinds or in different parts of the body, exist simultaneously, to recognise that they are distinct as soon as they are perceived, and to direct our attention at will to any one of them separately. Thus at any moment we can be separately conscious of what we see, of what we hear, of what we feel, and distinguish what we feel in a finger or in the great toe, whether pressure or a gentle touch, or warmth. So also in the field of vision. Indeed, as I shall endeavour to shew in what follows, we readily distinguish our sensations from one another when we have a precise knowledge that they are composite, as, for example, when we have become certain, by frequently repeated and invariable experience, that our present sensation arises from the simultaneous action of many independent stimuli, each of which usually excites an equally well-known individual sensation. This induces us to think that nothing can be easier, when a number of different sensations are simultaneously excited, than to distinguish them individually from each other, and that this is an innate faculty of our minds.
Thus we find, among others, that it is quite a matter of course to hear separately the different musical tones which come to our senses collectively, and expect that in every case when two of them occur together, we shall be able to do the like.
The matter is very different when we set to work at investigating the more unusual cases of perception, and at more completely understanding the conditions under which the above-mentioned distinction can or cannot be made, as is the case in the physiology of the senses. We then become aware that two different kinds or grades must be distinguished in our becoming conscious of a sensation. The lower grade of this consciousness, is that where the influence of the sensation in question makes itself felt only in the conceptions we form of external things and processes, and assists in determining them. This can take place without our needing or indeed being able to ascertain to what particular part of our sensations we owe this or that relation of our perceptions. In this case we will say that the impression of the sensation in question is perceived synthetically. The second and higher grade is when we immediately distinguish the sensation in question as an existing part of the sum of the sensations excited in us. We will say then that the sensation is perceived analytically.[21] The two cases must be carefully distinguished from each other.
Seebeck and Ohm are agreed that the upper partials of a musical tone are perceived synthetically. This is acknowledged by Seebeck when he admits that their action on the ear changes the force or quality of the sound examined. The dispute turns upon whether in all cases they can be perceived analytically in their individual existence; that is, whether the ear when unaided by resonators or other physical auxiliaries, which themselves alter the mass of musical sound heard by the observer, can by mere direction and intensity of attention distinguish whether, and if so in what force, the Octave, the Twelfth, &c., of the prime exists in the given musical sound.
In the first place I will adduce a series of examples which shew that the difficulty felt in analysing musical tones exists also for other senses. Let us begin with the comparatively simple perceptions of the sense of taste. The ingredients of our dishes and the spices with which we flavour them, are not so complicated that they could not be readily learned by any one. And yet there are very few people who have not themselves practically studied cookery, that are able readily and directly to discover, by the taste alone, the ingredients of the dishes placed before them. How much practice, and perhaps also peculiar talent, belongs to wine tasting for the purpose of discovering adulterations is known in all wine-growing countries. Similarly for smell; indeed the sensations of taste and smell may unite to form a single whole. Using our tongues constantly, we are scarcely aware that the peculiar character of many articles of food and drink, as vinegar or wine, depends also upon the sensation of smell, their vapours entering the back part of the nose through the gullet. It is not till we meet with persons in whom the sense of smell is deficient that we learn how essential a part it plays in tasting. Such persons are constantly in fault when judging of food, as indeed any one can learn from his own experience, when he suffers from a heavy cold in the head without having a loaded tongue.
When our hand glides unawares along a cold and smooth piece of metal we are apt to imagine that we have wetted our hand. This shews that the sensation of wetness to the touch is compounded out of that of unresisting gliding and cold, which in one case results from the good heat-conducting properties of metal, and in the other from the cold of evaporation and the great specific heat of water. We can easily recognise both sensations in wetness, when we think over the matter, but it is the above-mentioned illusion which teaches us that the peculiar feeling of wetness is entirely resolvable into these two sensations.
The discovery of the stereoscope has taught us that the power of seeing the depths of a field of view, that is, the different distances at which objects and their parts lie from the eye of the spectator, essentially depends on the simultaneously synthetical perceptions of two somewhat different perspective images of the same objects by the two eyes of the observer. If the difference of the two images is sufficiently great it is not difficult to perceive them analytically as separate. For example, if we look intently at a distant object and hold one of our fingers slightly in front of our nose we see two images of our finger against the background, one of which vanishes when we close the right eye, the other belonging to the left. But when the differences of distance are relatively small, and hence the differences of the two perspective images on the retina are so also, great practice and certainty in the observation of double images is necessary to keep them asunder, yet the synthetical perception of their differences still exists, and makes itself felt in the apparent relief of the surface viewed. In this case also, as well as for upper partial tones, the ease and exactness of the analytical perception is far behind that of the synthetical perception.
In the conception which we form of the direction in which the objects viewed seem to lie, a considerable part must be played by those sensations, mainly muscular, which enable us to recognise the position of our body, of the head with regard to the body, and of the eye with regard to the head. If one of these is altered, for example, if the sensation of the proper position of the eye is changed by pressing a finger against the eyeball or by injury to one of the muscles of the eye, our perception of the position of visible objects is also changed. But it is only by such occasional illusions that we become aware of the fact that muscular sensations form part of the aggregate of sensations by which our conception of the position of a visible object is determined.
The phenomena of mixed colours present considerable analogy to those of compound musical tones, only in the case of colour the number of sensations reduces to three, and the analysis of the composite sensations into their simple elements is still more difficult and imperfect than for musical tones. As early as 1686 R. Waller mentions in the Philosophical Transactions the reduction of all colours to the mixture of three fundamental colours, as something already well known. This view could in earlier times only be founded on sensations and experiments arising from the mixture of pigments. In recent times we have discovered better methods, by mixing light of different colours, and hence have confirmed the correctness of that hypothesis by exact measurements, but at the same time we have learned that this confirmation only succeeds within a certain limit, conditioned by the fact that no kind of coloured light exists which can give us the sensation of a single one of the fundamental colours with exclusive purity. Even the most saturated and purest colours that the external world presents to us in the prismatic spectrum, may by the development of secondary images of the complementary colours in the eye be still freed as it were from a white veil, and hence cannot be considered as absolutely pure. For this reason we are unable to shew objectively the absolutely pure fundamental colours from a mixture of which all other colours without exception can be formed. We only know that among the colours of the spectrum scarlet-red, yellow-green, and blue-violet approach to them nearer than any other objective colours.[22] Hence we are able to compound out of these three colours almost all the colours that usually occur in different natural bodies, but we cannot produce the yellow and blue of the spectrum in that complete degree of saturation which they reach when purest within the spectrum itself. Our mixtures are always a little whiter than the corresponding simple colours of the spectrum. Hence it follows that we never see the simple elements of our sensations of colour, or at least see them only for a very short time in particular experiments directed to this end, and consequently cannot have any such exact or certain image in our recollection, as would indisputably be necessary for accurately analysing every sensation of colour into its elementary sensations by inspection. Moreover we have relatively rare opportunities of observing the process of the composition of colours, and hence of recognising the constituents in the compound. It certainly appears to me very characteristic of this process, that for a century and a half, from Waller to Goethe, every one relied on the mixtures of pigments, and hence believed green to be a mixture of blue and yellow, whereas when sky-blue and sulphur-yellow beams of light, not pigments, are mixed together, the result is white. To this very circumstance is due the violent opposition of Goethe, who was only acquainted with the colours of pigments, to the assertion that white was a mixture of variously coloured beams of light. Hence we can have little doubt that the power of distinguishing the different elementary constituents of the sensation is originally absent in the sense of sight, and that the little which exists in highly educated observers, has been attained by specially conducted experiments, through which of course, when wrongly planned, error may have ensued.
On the other hand every individual has an opportunity of experimenting on the composition of two or more musical sounds or noises on the most extended scale, and the power of analysing even extremely involved compounds of musical tones, into the separate parts produced by individual instruments, can readily be acquired by any one who directs his attention to the subject. But the ultimate simple elements of the sensation of tone, simple tones themselves, are rarely heard alone. Even those instruments by which they can be produced, as tuning-forks before resonance chambers, when strongly excited, give rise to weak harmonic upper partials, partly within and partly without the ear, as we shall see in Chapters V. and VII. Hence in this case also, the opportunities are very scanty for impressing on our memory an exact and sure image of these simple elementary tones. But if the constituents to be added are only indefinitely and vaguely known, the analysis of the sum into those parts must be correspondingly uncertain. If we do not know with certainty how much of the musical tone under consideration is to be attributed to its prime, we cannot but be uncertain as to what belongs to the partials. Consequently we must begin by making the individual elements which have to be distinguished, individually audible, so as to obtain an entirely fresh recollection of the corresponding sensation, and the whole business requires undisturbed and concentrated attention. We are even without the ease that can be obtained by frequent repetitions of the experiment, such as we possess in the analysis of musical chords into their individual tones. In that case we hear the individual tones sufficiently often by themselves, whereas we rarely hear simple tones and may almost be said never to hear the building up of a compound from its simple tones.
The results of the preceding discussion may be summed up as follows:—
In the next chapter we shall give details of the relations of the upper partials to the quality of compound tones.